Perfect squares, an intriguing aspect of number theory, are integers that result from multiplying a whole number by itself. In this comprehensive article, we will delve into the definition, explore a list of perfect squares, examine a chart illustrating their patterns, and provide illustrative examples. Join us on this journey through the realm of perfect squares and discover the mathematical elegance they bring to the table.
Table of Contents
Definition of Perfect Squares
A perfect square is a number that can be expressed as the product of an integer multiplied by itself. In mathematical terms, it takes the form \(n \times n\), where n is an integer. The result is a number with distinct properties and fascinating mathematical patterns.
List of Perfect Squares:
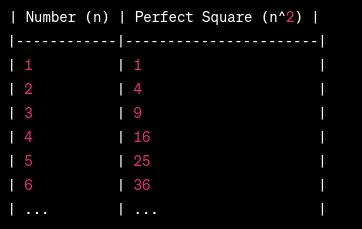
To provide a glimpse into the world of perfect squares, here’s a list showcasing some of these special numbers:
- 1 \((1^2)\)
- 4 \((2^2)\)
- 9 \((3^2)\)
- 16 \((4^2)\)
- 25 \((5^2)\)
- 36 \((6^2)\)
…
Chart of Perfect Squares:
Visualizing the patterns of perfect squares can enhance understanding. Consider the following chart:
 Perfect Squares Examples
Perfect Squares Examples
- \(4^2\) =16: The square of 4 is 16.
- \(7^2\) = 49 : The square of 7 is 49.
- \(10^2\) =100: The square of 10 is 100.
- \(15^2\) = 225: The square of 15 is 225.
- \(20^2 \) = 400: The square of 20 is 400.
- \(25^2\) = 625: The square of 25 is 625.
- \(8^2 \) = 64: The square of 8 is 64.
- \(12^2\) =144: The square of 12 is 144.
- \(18^2\) = 324: The square of 18 is 324.
- \(22^2\) = 484: The square of 22 is 484.
FAQs
Q1: What is a perfect square?
- A1: A perfect square is a number that can be expressed as the product of an integer multiplied by itself. In mathematical terms, it is in the form \(n \times n\), where is an integer.
Q2: Can negative numbers be perfect squares?
- A2: No, perfect squares are non-negative integers. The square of any real number, whether positive or negative, results in a non-negative value.
Q3: How can I recognize a perfect square?
- A3: A perfect square is often recognizable by the fact that its square root is a whole number. For example, the square root of 25 is 5, making 25 a perfect square.
Q4: Are all integers perfect squares?
- A4: No, not all integers are perfect squares. Only numbers that can be expressed as the square of an integer are perfect squares.
Q5: What is the significance of perfect squares in mathematics?
- A5: Perfect squares have applications in various mathematical concepts, including geometry, algebra, and number theory. They are fundamental in understanding patterns and relationships between numbers.
Q6: Can fractions or decimals be perfect squares?
- A6: In their standard form, perfect squares are whole numbers. Fractions or decimals are generally not considered perfect squares.
Q7: How are perfect squares used in real-world applications?
- A7: Perfect squares are used in real-world applications such as measuring areas in construction, calculating distances in physics, and in various mathematical modeling scenarios.
Q8: Are all square numbers perfect squares?
- A8: Yes, all square numbers are perfect squares. A square number is simply the result of multiplying a number by itself.
Q9: How many perfect squares are there between 1 and 20?
Between 1 and 20, the perfect squares are:
- 1\(\times 1 = 1\)
- 2\( \times 2 = 4\)
- 3\( \times 3 = 9\)
- 4 \(\times 4 = 16\)
So, there are four perfect squares between 1 and 20.